Sunday Times Teaser 3073 – Snookered

by BRG on August 14, 2021

by Nick MacKinnon

Published Sunday August 15 2021 (link)

The playing surface of a snooker table is a twelve-foot by six-foot rectangle. A ball is placed at P on the bottom cushion (which is six feet wide) and hit so it bounces off the left cushion, right cushion and into the top-left pocket.

Now the ball is replaced at P and hit so it bounces off the left cushion, top cushion and into the bottom right pocket, after travelling 30% further than the first shot took. The ball always comes away from the cushion at the same angle that it hits the cushion.

How far did the ball travel on the second shot?

From → Uncategorized

One Comment Leave one →

Brian Gladman permalink

We can treat the paths of the balls as the equivalent of rays of light reflecting on mirrors as illustrated here:

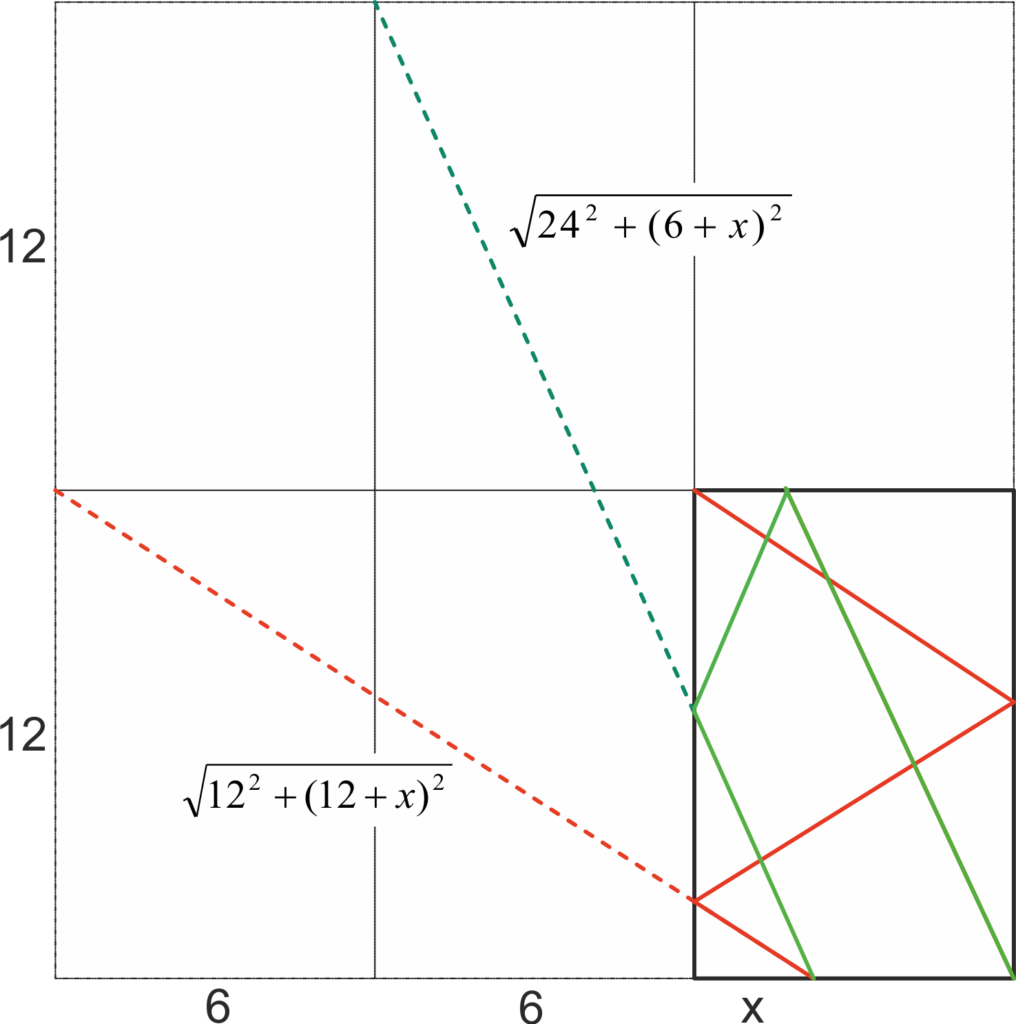
We are told that the second path is 30% longer than the first so we have:\[13^2(12^2+(12+x)^2)=10^2(24^2+(6+x)^2)\]which after simplification becomes:\[69x^2+2856x-12528 = 0\]with the factors\[(23x+1044)(3x-12)=0\]With its only positive root, \(x=4\), this gives the path lengths as 20 and 26 feet (assuming a zero diameter ball).

The first and second paths involve 4 times (3, 4, 5) and 2 times (5, 12, 13) Pythagorean triangles respectively.


from math import isqrt

#             12                   6     x
#        +----------+         +-----+--+--+-----+
#        |          |         |      \ | /      |
#        |         /|         |       \|/       |
#        |        / |x      12|        +        |
#        +-------+--+         |       /|        |
#        |      /   |         |      / |        |
#        |     /    |6        +-----+--+--------+
#        |    /     |         |    /   |        |
#        +---+------+         |   /    |        |
#        |  /       |       12|  /     |        |
#        | /        |6        | /      |        |
#        |/         |         |/       |        |
#        +----------+         +--------+--------+
#             12
#
#   f^2 = 12^2 + (12+x)^2    g^2 = 24^2 + (6+x)^2

# the distance of the starting position from the bottom
# left corner along the bottom edge of the table, which 
# is assumed to be an integer as this is a teaser (also
# a zero diameter ball is assumed)
for x in range(7):
  
  # the first path length
  f2 = 12 ** 2 + (12 + x) ** 2
  f = isqrt(f2)
  if f * f != f2:
    continue

  # the second path length   
  g2 = 24 ** 2 + (6 + x) ** 2
  g = isqrt(g2)
  if g * g != g2:
    continue
  
  # look for the second path length
  # equal to 13 / 10 times the first
  if 13 * f == 10 * g:
    print(f"Shot lengths: {f} feet (1st), {g} feet (2nd).")

from math import isqrt

# 12 6 x

# +----------+ +-----+--+--+-----+

# | | | \ | / |

# | /| | \|/ |

# | / |x 12| + |

# +-------+--+ | /| |

# | / | | / | |

# | / |6 +-----+--+--------+

# | / | | / | |

# +---+------+ | / | |

# | / | 12| / | |

# | / |6 | / | |

# |/ | |/ | |

# +----------+ +--------+--------+

# 12

# f^2 = 12^2 + (12+x)^2 g^2 = 24^2 + (6+x)^2

# the distance of the starting position from the bottom

# left corner along the bottom edge of the table, which

# is assumed to be an integer as this is a teaser (also

# a zero diameter ball is assumed)

for x in range(7):

# the first path length

f2 = 12 ** 2 + (12 + x) ** 2

f = isqrt(f2)

if f * f != f2:

continue

# the second path length

g2 = 24 ** 2 + (6 + x) ** 2

g = isqrt(g2)

if g * g != g2:

continue

# look for the second path length

# equal to 13 / 10 times the first

if 13 * f == 10 * g:

print(f"Shot lengths: {f} feet (1st), {g} feet (2nd).")

Sunday Times Teaser 3073 – Snookered

by Nick MacKinnon

Published Sunday August 15 2021 (link)

Leave a Reply Cancel reply

Recent Posts

Recent Comments

Archives

Categories

Meta